

That is, unless the subset has already been verified to be a subspace: see this important notebelow. In order to verify that a subset of Rnis in fact a subspace, one has to check the three defining properties. Making subsets of vector spaces is kind of like removing parts of a vector space such that the remaining part keeps the structure of a vector space. A subspace is a subset that happens to satisfy the three additional defining properties. Linear combinations and linear independence A linear combination is a sum of scalar multiples of vectors. will give on set 0 and you have to prove it is subspace or not 14.

linearly independent and linearly dependent sets 12.
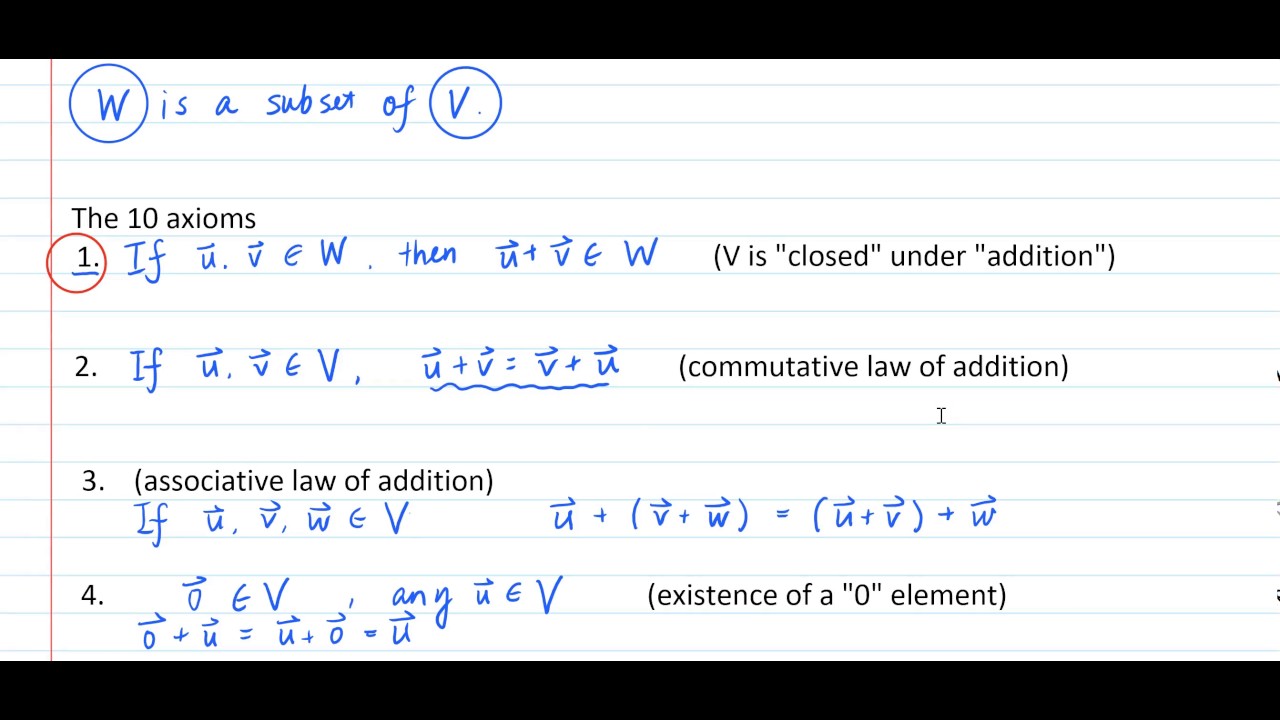
What is Linear transformation : mapping between two vector spaces are. The span of a vector set is the smallest vector space that includes that vector set. Subspaces A subset of a vector space is a subspace if it is non-empty and, using the restriction to the subset of the sum and scalar product operations, the subset satisfies the axioms of a vector space. View linear algebra viva questions.docx from MATH 2 564A at U.E.T Taxila. A subspace of a vector space is a subset of the "bigger" vector space such that it is also a vector space (basically a smaller set that doesn't lose the structure of the bigger set, that is a vector space structure).Īnd when we're talking about the span of a vector set, we're relating a vector space to a "smaller" set of vectors that could or not be also a vector space. When we're talking about subspaces we're relating them to a bigger vector space. It must be closed under scalar multiplication: if v S v. It must be closed under addition: if v 1 S v 1 S and v 2 S v 2 S for any v 1, v 2 v 1, v 2, then it must be true that (v 1 + v 2) S (v 1 + v 2) S or else S S is not a subspace. But what makes them different from each other is how they relate to other things. The formal definition of a subspace is as follows: It must contain the zero-vector. A span of a vector set and a subset have the same structure, they're both vector spaces.


 0 kommentar(er)
0 kommentar(er)
